A Cellular Automaton Simulation Model Considering Spatial-temporal Distribution for Mixed Bicycle Flows
-
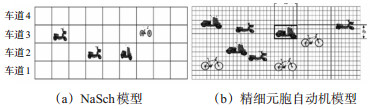
摘要: 针对非机动车交通流中传统元胞自动机模型主观定义时空参数,粗略划分自行车虚拟车道,导致仿真精度偏差的问题,构建了精细元胞自动机模型。基于NaSch模型的更新规则,考虑二维空间内异质自行车间的错位冲突及动态换道行为特征,细化了模型网格密度及模拟时间步长。产生换道需求的自行车可以换至满足安全侧向换道条件及前行需求条件的横向位置,随后综合考虑各位置前向及侧向间隙选取最优的目标位置执行换道。在周期边界条件下,量化时空参数取值对混合自行车交通流仿真结果的影响。在镇江市正东路开展观测实验,并基于实测数据获得轨迹时空图,在宏观与微观层面验证模型的可靠性。结果表明:网格密度及时间步长取值对仿真流量影响显著,仿真流量与纵向网格密度呈正相关,与横向网格密度呈负相关,而全局网格密度的影响反映了横向与纵向网格密度的复合效应;当道路占有率为0.1时,仿真流量几乎不受时间步长的影响,而当道路占有率为0.3,0.5和0.7时,仿真流量随着时间步长的增大先增加后减小;自行车适度换道可以提高道路通行能力,但换道过于频繁则会导致交通拥堵,不同道路占有率条件下的轨迹时空特征存在明显差异,自行车流越密集,走停现象越显著;当全局网格密度为5,时间步长为0.5 s时,模拟与实测的交通流量平均绝对百分比误差为14.84%,此时拟合效果最优。Abstract: Traditional cellular automata(CA)models provide inaccurate simulation results in modeling non-motorized traffic flow, due to the fact that they subjectively define spatial-temporal parameters and roughly represent bicycle lanes. With this, an improved CA model is proposed in this paper. Specifically, the grid density and time step of the proposed model are upgraded based on the updating rules of a NaSch model, which considers the conflict between heterogeneous bicycles and the dynamic lane-changing behavior in a two-dimensional space. In the proposed model, bicycles that need to make lane-changing can change to a lateral position which meets the condition of safe lateral and the forward movement. The bicycle can change lanes to the optimal position considering both the forward and lateral distance of each position. In addition, the influence of different spatial-temporal parameters on simulation results is quantified under the period boundary condition. Data from Zhengdong Road in Zhenjiang is obtained, and the spatial-temporal diagrams of trajectories are generated and with which the reliability of the proposed model is verified at both the macro and micro levels. Study results are supportive for the following conclusions. First, grid density and time step have a significant impact on the simulated flows and they are positively correlated with the longitudinal grid density but negatively correlated with the lateral grid density, and their global grid density is the compound effect of the two densities. Second, the flow is almost unaffected by the size of time step when the occupancy rate is around 0.1, but when the occupancy rates is around 0.3, 0.5, or 0.7, the bicycle flow shows similar trend that first increase and then decrease with the increment of time step. Third, moderate lane-changing behavior of bicycles can improve road capacity, while frequent lane-changing behavior would lead to congestion. Significant differences in the spatial-temporal diagrams of trajectories are found under different occupancy conditions, and bicycle flows with a high density would lead to stop-and-go condition. Fourth, when the global grid density is 5 and the time step is 0.5 s, the accuracy is highest, where the mean absolute percentage error(MAPE)between simulated results and observed data is only 14.84%.
-
表 1 基于元胞自动机的自行车交通流建模参数
Table 1. Parameters of bicycle simulation based on CA model
文献 年份 模型 车辆类型 元胞长度/m 车辆尺寸/m 占用元胞数 时间步/s 邝先验等[12] 2015 改进的NaSch模型 电动自行车 2×0.9 2×0.9 1×1 1 电动三轮车 2×1.8 1×2 邝先验等[13] 2016 改进的NaSch模型 人力自行车 2×1 2×1 1×1 1 电动自行车 2×1 1×1 Tang等[8] 2018 改进的NaSch模型 人力自行车 0.75×0.75 1.5×0.75 2×1 1 行人 1×1 2×2 Liu等[11] 2015 改进的NaSch模型 人力自行车 0.5×0.5 2×1 4×2 1 摩托车 3×1 6×2 机动车 7×2.5 14×5 人力自行车 2×0.8 1×1 Li等[10] 2020 改进的NaSch模型 电动自行车 2×0.8 2×0.8 1×1 1 机动车 6×2.4 3×3 冯雪等[14] 2016 改进的NaSch模型 机动车 1×1 7×动态宽度 7×动态宽度 1 非机动车 2×1 2×1 Jin等[19] 2015 多值元胞自动机模型 人力自行车 2×* 2×* 1 1 电动自行车 2×* 1 注:*号表示相应参数无具体数值说明。 表 2 全局网格密度n
Table 2. Global grid density n
n cell size/m2 ny nx 1 0.9×0.9 1 2 2 0.45×0.45 2 4 3 0.3×0.3 3 6 4 0.22×0.22 4 8 5 0.18×0.18 5 10 6 0.15×0.15 6 12 -
[1] 杨龙海, 张春, 仇晓赟, 等. 车辆跟驰模型研究进展[J]. 交通运输工程学报, 2019, 19(5): 125-138. https://www.cnki.com.cn/Article/CJFDTOTAL-JYGC201905015.htmYANG L H, ZHANG C, CHOU X Y, Research progress on car-following models[J]. Journal of Traffic and Transportation Engineering, 2019, 19(5): 125-138. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JYGC201905015.htm [2] SUTOMO H. Appropriate saturation flow at traffic signals in Javanese cities a modelling approach[J]. Chemistry-A European Journal, 1992, 15(4): 885-900. [3] 李星星, 马健霄, 徐佳逸. 非机动车跟驰模型的研究[J]. 交通运输工程与信息学报, 2012, 10(1): 115-120. https://www.cnki.com.cn/Article/CJFDTOTAL-JTGC201201019.htmLI X X, MA J X, XU J Y. Study on non-motorized vehicle-following model[J]. Journal of Transportation Engineering and Information, 2012, 10(1): 115-120. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JTGC201201019.htm [4] 周泱, 周竹萍, 徐永能, 等. 交叉口绿闪信号行人过街行为模型[J]. 交通信息与安全, 2018, 36(1): 74-80. https://www.cnki.com.cn/Article/CJFDTOTAL-JTJS201801011.htmZHOU Y, ZHOU Z P, XV Y N, et al. A model for crossing behaviors of pedestrians at intersections during flashing green signals[J]. Journal of Transport Information and Safety, 2018, 36(1): 74-80. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JTJS201801011.htm [5] 杨文彦, 张希, 陈浩, 等. 基于社会力的自动驾驶汽车行人轨迹预测模型[J]. 公路交通科技, 2020, 37(8): 127-135. https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK202008016.htmYANG W Y, ZHANG X, CHENG H, et al. A model of pedestrian trajectory prediction for autonomous vehicles based on social force[J]. Journal of Highway and Transportation Research and Development, 2020, 37(8): 127-135. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK202008016.htm [6] 王占中, 赵利英, 焦玉玲, 等. 信号交叉口自行车和行人混合交通流社会力模型[J]. 吉林大学学报(工学版), 2018, 48(1): 89-97. https://www.cnki.com.cn/Article/CJFDTOTAL-JLGY201801011.htmWANG Z Z, ZHAO L Y, JIAO Y L, et al. Social force model of pedestrain-bike mixed flow at signlized crosswalk[J]. Journal of Jilin University(Engineering and Technology Edition), 2018, 48(1): 89-97. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JLGY201801011.htm [7] NAGEL K, SCHRECKENBERG M. A cellular automaton model for freeway traffic[J]. Journal De Physique I, 1992, 2(12): 221-2229. [8] TANG T Q, RUI Y X, ZHANG J, et al. A cellular automation model accounting for bicycle's group behavior[J]. Physica A: Statistical Mechanics and its Applications, 2018(492): 1782-1797. [9] 文夏梅, 傅立平, 钟鸣, 等. 城市混合交通共享街道驾驶行为及行人过街安全研究[J]. 交通信息与安全, 2020, 38(5): 12-19. https://www.cnki.com.cn/Article/CJFDTOTAL-JTJS202005005.htmWEN X M, FU L P, ZHONG M, et al. Analysis of driving behaviors and pedestrian crossing safety on urban roadway with mixed traffic flow[J]. Journal of Transport Information and Safety, 2020, 38(5): 12-19. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JTJS202005005.htm [10] LI Y X, NI Y, SUN J, et al. Modeling the illegal lane-changing behavior of bicycles on road segments: Considering lane-changing categories and bicycle heterogeneity[J]. Physica A: Statistical Mechanics and its Applications, 2020(541): 123302. [11] LIU M F, XIONG S W. A refined and dynamic cellular automaton model for pedestrian-vehicle mixed traffic flow[J]. International Journal of Modern Physics C, 2016, 27(5): 1650053. [12] 邝先验, 吴赟, 曹韦华, 等. 城市混合非机动车流的元胞自动机仿真模型[J]. 广西师范大学学报(自然科学版), 2015, 33(1): 7-14. https://www.cnki.com.cn/Article/CJFDTOTAL-GXSF201501002.htmKUANG X Y, WU Y, CAO W H, et al. Cellular automata simulation model for urban mixed non-motor vehicle flow[J]. Journal of Guangxi Normal University: Natural Science Edition, 2015, 33(1): 7-14. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GXSF201501002.htm [13] 邝先验, 曹韦华, 吴赟. 考虑混入逆行车辆的非机动车流元胞自动机模型[J]. 系统仿真学报, 2016, 28(2): 268-274. https://www.cnki.com.cn/Article/CJFDTOTAL-XTFZ201602002.htmKUANG X Y, WU Y, CAO W H, et al. Cellular automata model of non-motor vehicle flow considering reverse vehicles[J]. Journal of System Simulation, 2016, 28(2): 268-274. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XTFZ201602002.htm [14] 冯雪, 王喜富. 考虑自行车流特性的机非混合交通流元胞自动机仿真[J]. 公路交通科技, 2016, 33(3): 132-137. https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK201603022.htmFENG X, WANG X F. Simulation of mixed traffic flow by cellular automaton considering bicycle flow characteristics[J]. Journal of Highway and Transportation Research and Development, 2016, 33(3): 132-137. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK201603022.htm [15] NISHINARI K, TAKAHASHI D. A new deterministic CA model for traffic flow with multiple states[J]. Journal of Physics A: Mathematical and General, 1999, 32(1): 93-104. [16] NISHINARI K, TAKAHASHI D. Multi-value cellular automaton models and metastable states in a congested phase[J]. Journal of Physics A: Mathematical and General, 2000(33): 7709. [17] 张兴强, 汪滢, 胡庆华. 交叉口混合交通流元胞自动机模型及仿真研究[J]. 物理学报, 2014, 63(1): 90-97. https://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201401011.htmZHANG X Q, WANG Y, HU Q H. Research and simulation on cellular automaton model of mixed traffic flow at intersection[J]. Acta Physica Sinica, 2014, 63(1): 90-97. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201401011.htm [18] XUE S Q, JIA B, JIANG R, et al. An improved Burgers cellular automaton model for bicycle flow[J]. Physica A: Statistical Mechanics and its Applications, 2017(487): 164-177. [19] JIN S, QU X, XU C, et al. An improved multi-value cellular automata model for heterogeneous bicycle traffic flow[J]. Physics Letters A, 2015, 379(39): 2409-2416. [20] 夏亮, 郭廷龙, 刘仰, 等. 基于多值CA模型的两轮车加速过程建模与分析[J]. 公路交通科技, 2017, 34(1): 112-119. https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK201701017.htmXIA L, GUO Y L, LIU Y, et al. Simulation and analysis of two-wheel vehicle acceleration based on multi-value CA model[J]. Journal of Highway and Transportation Research and Development, 2017, 34(1): 112-119. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GLJK201701017.htm [21] 严巧兵. 基于社会力模型的非机动车混合流仿真研究[D]. 北京: 北京建筑大学, 2020.YAN Q B. Research on mixed non-motorized flow simulation based on social force model[D]. Beijing: Beijing University of Civil Engineering and Architecture, 2020. (in Chinese) -





 下载:
下载: